Scattering Cross Section Pdf

The differential cross section dσ dθ with respect to the scattering angle is the number of scatterings between θand θ dθ per unit flux per unit range of angle i e.

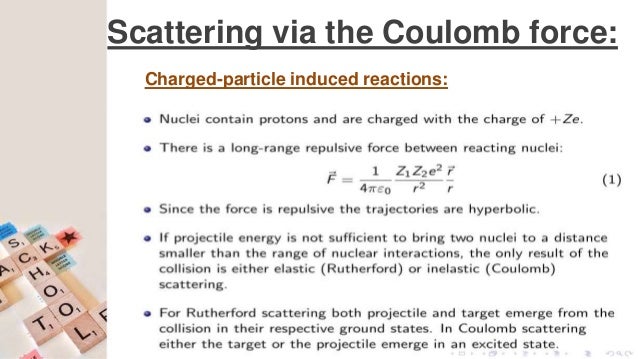
Scattering cross section pdf. The scattering cross section is a vital operator to understand the physical interaction between low energy photons and the physical properties of the. For the case of scattering by a free electron so that q and m assume the values appropriate for an electron the numerical result is. Using the expression for the polar.

This expression gives the cross section for the scattering of an unpolarized plane electromagnetic wave by a free charged particle and is known as the thomson scattering cross section denoted by σ t. Dσ dθ dn θ fdθ π d2 4 cos θ 2 sin 3 θ 2. Cross sectional area of an object with a linear extent on the order of 10 12 cm.

Experimentally determine the scattering cross section by knowing the density of targets the number of incident particles and the number of scattered particles. It is more usual to quote the differential cross section with respect to a given solid angle. Quantize of scattering theory.

Calculate the di erential and total scattering cross sections d d and tot as a. The scattering cross section was defined in section 3 8 1 as the equivalent area of the incident beam that intercepts the same energy as that scattered so the rayleigh scattering cross section is σsca r4π 0 α2k4 1 cos2 θ 2 e 0 dω s e0 8 3 πα2k4 5 19 where the axes have been chosen so that θ θ. Corrections for the measure ments.

In order to make use of this idea for a realistic. In classical scattering the idea is that if we take a cross section of the incoming particle beam and divide this cross section up into little regions of area d then a particle that crosses the area element d will always classically scatter into an element of solid angle dw. Given the scattering potential v r.

Ering the differential cross section. Of course this formula would have limited utility if it could only describe the scattering of hard spheres. Cross section from the differential we can obtain the total cross section by integrating over all solid angles σ dσ dω dω 2π 0 dφ π 0 dθ sin θ dσ dω the cross section which typically depends sensitively on energy of incoming particles has dimensions of area and can be separated into σ elastic σ.